Looking for a Word, Math Related...

[-K2-]

I'm looking for a word--if one exists--that is used to describe coincidences in number and equation sets. I get that there is nothing mystical about what I'm finding, I'm just looking for a term for such things. As an example without going into the why, I have a condition where the first group of numbers are sums generated by a particular shape subdivided 9 times (each section numbered, then added, excluding one section), and then 3 common modifiers:

I suppose I might as well add the subdivided+modifier shape:

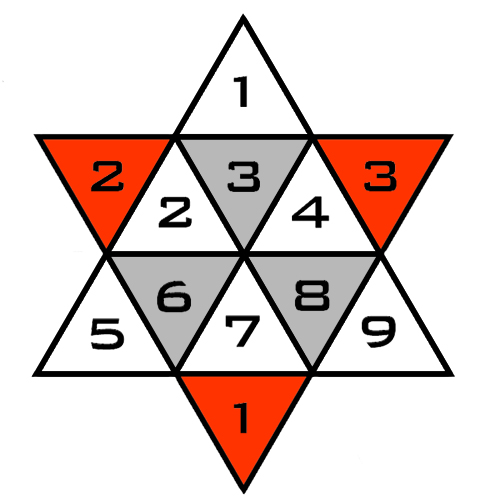
Sum: +1, +2, +3
44 : 45 - 46 - 47
43 : 44 - 45 - 46
42 : 43 - 44 - 45
41 : 42 - 43 - 44
40 : 41 - 42 - 43
39 : 40 - 41 - 42
38 : 39 - 40 - 41
37 : 38 - 39 - 40
36 : 37 - 38 - 39

As you can see the groupings result in diagonal lines of common numbers. If I then take the first column and add rows 1+9, then 2+8, then 3+7, etc. they each equal 80. If I then take corner to corner of the 1+9 row, middle, opposite corner...then do the same with rows 2+8, 3+7, etc. in each case I get 84.

44+36+80 / 45+39=84, 46+38=84, 47+37=84
43+37=80 / 44+40=84, 45+39=84, 46+38=84
42+38=80 / 43+41=84, 44+40=84, 45+39=84
41+39=80 / 42+42=84, 43+41=84, 44+40=84
40+40=80 / 41+43=84, 42+42=84, 43+41=84

That's enough to describe the condition, simply know with numerous base variables based off this one shape subdivided and then with three modifiers used, I get all sorts of coincidental results like this. They actually mean nothing--which is somewhat the point--but I'm wondering if there is a term to describe (or class) such outcomes. Besides kooky, nutty, off your meds, whacko, deranged, de-foiled, etc.

Thanks for your help!

K2

 

[Danny McG]

 

[ginny]

I think that's called mathematical coincidence; which sounds a bit trite(I know); however that's what it is.

 

[Ori Vandewalle]

When people invest meaning in meaningless mathematical coincidences, that gets called numerology. Not sure if that's what you're going for, though.

 

[Daysman]

Yup, 47 + 37 = 84...

Reminds me of the Bible code... did a quick wiki and a few links out found apophenia?

I need food.

 

[-K2-]

Well thanks @ginny , @Ori Vandewalle , @Daysman , and even @dannymcg for his knuckle-dragging suggestion

'Mathematical coincidence' was what I knew it to be, but I wondered if there was a different--perhaps psychological term with such an obsession--applied to it. As said, by coincidence, there are a significant number of coincidences I've recognized by the set number groups and a few that follow a track then fail (which I've labeled as 'bit-traps').

Long story short, with an alphabet based off number combinations--IN THE STORY--those coincidences will be recognized by the developer as just that, yet posed to students as proof of the alphabet's validity. So, as folks question that and search out coincidences, in truth they're simply learning the alphabet and the language's spelling rules, fixing the alphabet in stone for the populace.

K2